If sinA=5/13 for angle A in Quadrant I, find tan2A asked Nov 26, 13 in TRIGONOMETRY by skylar Apprentice doubleangle;1) 2) 3) 4) 4 Which angle is coterminal with an angle of 125°?1) –125° 2) –235° 3) 235° 4) 425° 5 Which angle has the same terminal side as an angle of 155°?

Rectangular Coordinate System
How to determine angle quadrant
How to determine angle quadrant-May 05, 21 · Determine the quadrants 0 to π/2 first quadrant, so reference angle = angle, π/2 to π second quadrant, so reference angle = π angle, π to 3π/2 third quadrant, so reference angle = angle π, 3π/2 to 2π fourth quadrant, so reference angle = 2π angle 10π/9 is a bit more than π, so it lies in the third quadrantOct 21, 18 · if sinx=7/5 and angle x is in quadrant 2 and cos y=12/13 and angle y is in quadrant 1 find sin (xy) asked Nov 26, 13 in TRIGONOMETRY by harvy0496 Apprentice doubleangle;



Rectangular Coordinate System
S 25 24 (3) (4) so Co) In which quadrant doesThanks Found 2 solutions by stanbon, Alan3354Other Special Angles We can also find the exact trigonometric functional values of a few other angles that relate to the angles θ = π/6, θ = π/4, and θ = π/3 in certain ways To look at these other angles, we introduce the concept of a reference angle, denotated as θ'
Sin π 3 = 3 2 2 π 3 is in quadrant II and sine is positive in quadrant II, so sin 2 π 3 = 3 2 13 π 4 is not between 0 and 2π, so begin by finding a coterminal angle between 0 and 2π 13 π 42 π = 13 π 48 π 4 = 5 π 4 Find the reference angle 5 π 4 is in quadrant III and closest xaxis angle is π 5 π 4π = 5 π 44 π 4 = π 4(1) 685 (2) 1291 (3) 1697 (4) 28 ase 12 If is an angle dravm in the standard position with its terminal ray landing in the fourth quadrant and csc a) = —5 , then which of the following is the exact value of cos (a) ?1) −5° 2)−155° 3)25° 4)335° 6 Which angle does not terminate in Quadrant IV when drawn on a unit circle in standard position?
If tanA=2/3 and sinB=5/7 and angles A and B are in Quadrant 1 fine the value of tan(AB)?Section_34_Notesdocx Section 34 1 2 Trig Equations including Factoring 9 sin2 \u0398 \u13 4 = 0 2 cos2 \u0398 3 cos \u0398 1 = 0 Quadrant Quadrant Angle AngleFind the values of the six trigonometric ratios for each angle below 1) 180° 2) 15π 3) 450° 4) 360° 5) 8π Calculator Solutions Input each ratio using the trigonometric keys Enter one ratio per line Add the degree symbol if the given angle is measured in degrees Otherwise, tap the DEG button to swift to RAD and add the π symbol



1 5 Plotting Points On A Coordinate Grid Gruending Math 6


If Tan 8 Is Positive And Sin 8 Is Negative In Which Quadrant Does 8 Lie Quora
Sal first finds the direction angle of a vector in the first quadrant, then moves onto a trickier one in the second quadrant to solve a for theta we could just say that theta is equal to the inverse tangent sometimes people say arctangent of 4/3 of 4 over 4 over 3 and let's evaluate this and I'm going to get my calculator out to do it so IThen $\tan^{1}\left({4\over3}\right) \approx 53^\circ$, which is in quadrant I However, this does not line up with our intuition of where this vector should land Hence, we may add $180^\circ$ to this degree measure to give us the correct angle measure which will land in quadrant III, since $53^\circ 180^\circ = 233^\circ$Namaste to all Friends, This Video Lecture Series presented By VEDAM Institute of Mathematics is Useful to all student



Sum And Difference Identities Precalculus Ii
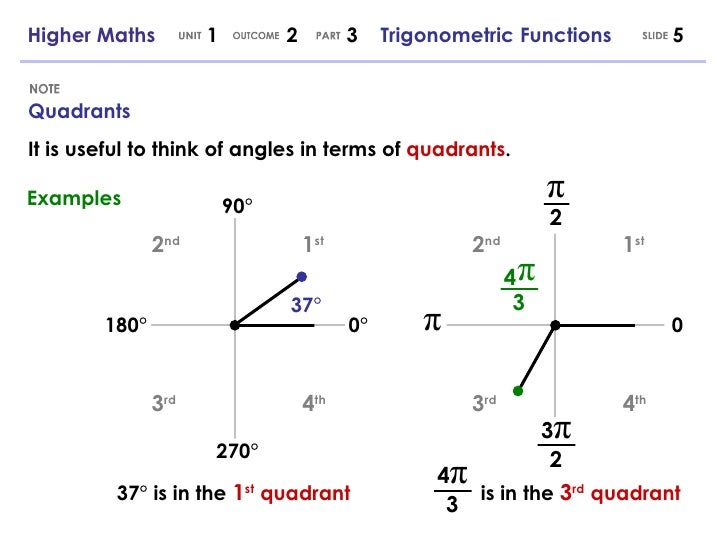


Higher Maths 1 2 3 Trigonometric Functions
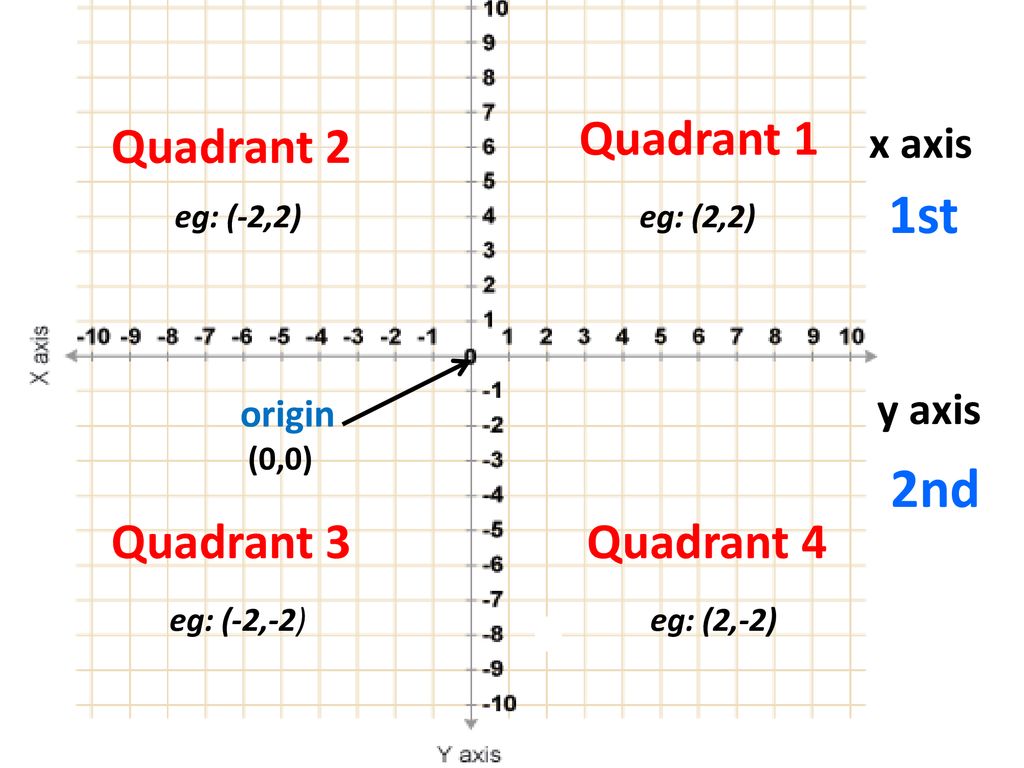
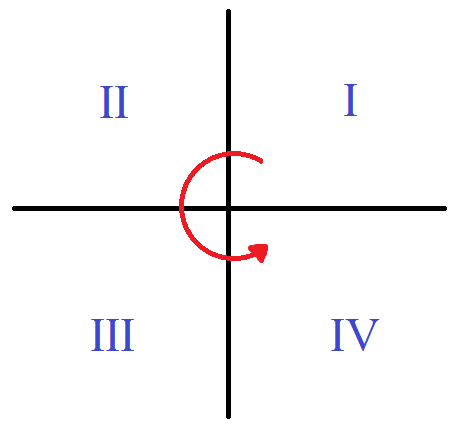
4 and 3 in conjunction with the CAST rule to determine the value and the sign J GarvinExact Values of Trigonometric Ratios Slide 6/16 Using the 12p 3 triangle, the opposite side to 3 has aQuadrant In mathematics, a quadrant is one of the four sections of a rectangular coordinate plane The quadrants are separated by the x and yaxes and are labeled I through IV, starting in the upper right quadrant and going counterclockwise, as shown in the figure below(1) I (3) III Which of the following could not be the value of the cosine of an angle?


Coordinate Geometry Gmat Math Study Guide
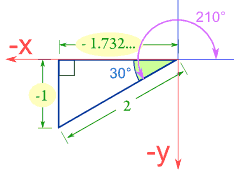


Sine Cosine And Tangent In Four Quadrants
Evaluating Trigonometric Functions Using Reference Angles 1 Determine the reference angle associated with the given angle 2 Evaluate the given trigonometric function of the reference angle 3 Affix the appropriate sign determined by the quadrant of the terminal side of the angle in standard position Example 13 Evaluate the following aHow to find all six trigonometric function values given one value and the quadrantThis video is provided by the Learning Assistance Center of Howard CommuniDraw a 37° angle in standard position together with a unit circle Use the triangle below to find the x any ycoordinates of the point of intersection of the terminal side and the circle Compute and Confirm that they are equal to and Draw the 37° angle in standard position The unit circle triangle is similar to the 345 right triangle
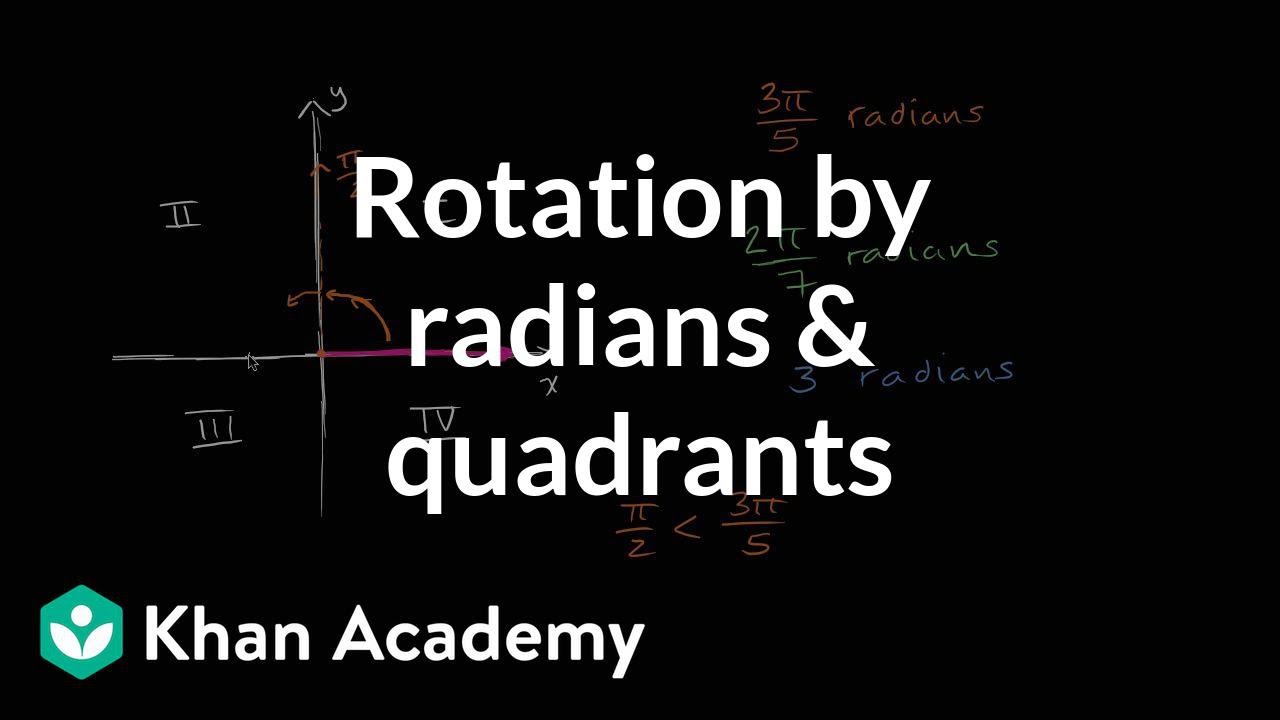


Radian Angles Quadrants Video Radians Khan Academy


In Which Quadrant Does 90 Degrees Lie Quora
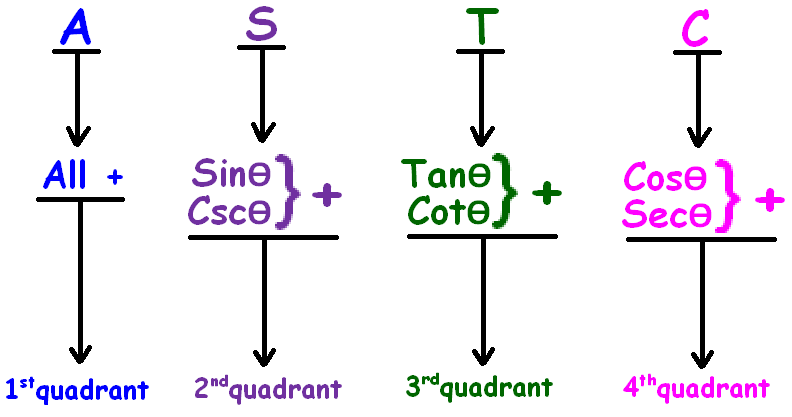
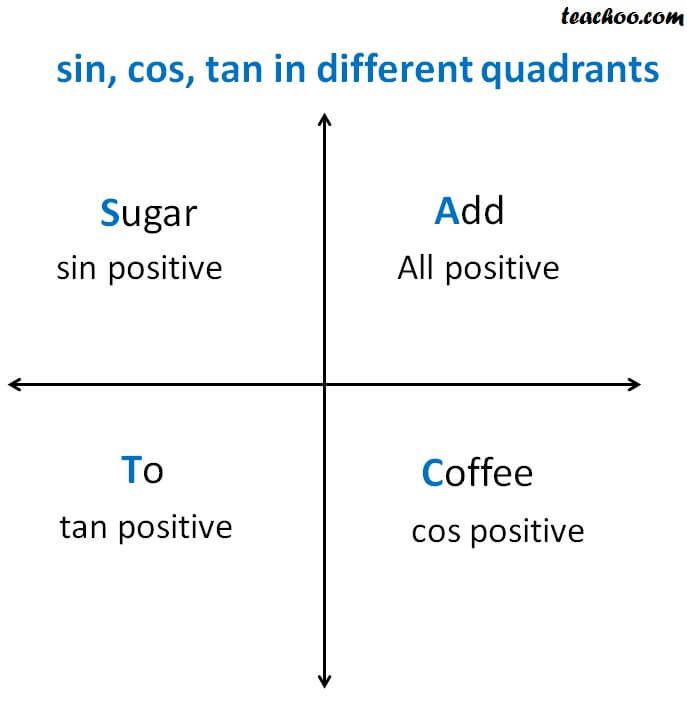
Apr 03, 16 · All the trig functions are positive in Quadrant 1 Sine and cosecant are positive in Quadrant 2, tangent and cotangent are positive in Quadrant 3, and cosine and secant are positive in Quadrant 41 If cos x = and x is a fourthquadrant angle, evaluate sin 2x These are my answer choices *sqrt(2) *1 *1 2 If θ is a firstquadrant angle in standard position with P(u, v) = (3, 4), evaluate tan θ Answer choices *1/4 *1/2 *2/3 Please explain with lots of steps!(1) 4 5 (3



Content The Four Quadrants
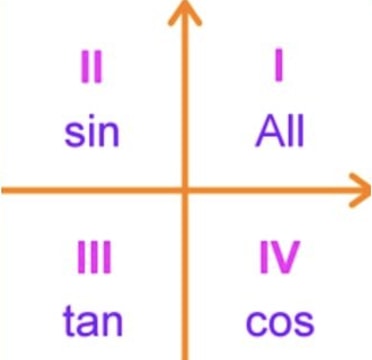


What Is All Students Take Calculus In Trig Studypug
1 p 3 2 = 1 p 3 (d) csc 5ˇ 4 Solution The angle 5ˇ 4 will fall in quadrant 2 with a reference angle of r = ˇ 4 Using either a special right triangle or the unit circle, we nd that csc 5ˇ 4 = csc ˇ 4 = 1 sin ˇ 4 = 1 p1 2 = p 2 (e) sec 48ˇ 3 Solution The angle 48ˇ 3 = 16ˇis a quadrantal angle that is coterminal to 2ˇ Thus, sec 48ˇ 3 = sec(16ˇ) = 1 cos16ˇ = 1 cos2ˇ = 1 1 = 1 (f) cos 11ˇ 3(1) 1 (3) 210 (2) 60 (4) 240 3 A rotation angle, drawn in standard position, measures 10 In which quadrant does its terminal ray lie?Apr 22, 21 · Since, 1 cycle is 2π radians, so it is a bit less than two cycles but more than 3/2 = 15 This angle thus lies in the fourth quadrant between 3π/2 and 2π radians Now, as we know, for fourth quadrant Reference Angle = 2 π – Given Angle, here given angle = 16/9 π radians = 2 π – 16/9 π = 2



Trigonometric Ratios Solutions Examples Videos


Cast Rule Mathonline
2 Degrees to Quadrants = 80 Degrees to Quadrants = 0 3 Degrees to Quadrants = 90 Degrees to Quadrants = 1 4 Degrees to Quadrants = 100 Degrees to Quadrants = 5 Degrees to Quadrants = 0 Degrees to Quadrants = 6 Degrees to Quadrants = 300 Degrees to Quadrants = 7 Degrees toCircle Which of the follo n is closest to the tangent of this angle?The angle measured is the sum of the angle indicated by the position of the transom and the angle measured on the scale on the arc The instrument that is now identified with Davis is shown in Figure 3 This form evolved by the mid17th century The quadrant


Astc Formula


How To Identify The Quadrant Of An Angle Quora
If θ is in quadrant II and cos θ = − 2/3The coordinate plane is divided into four regions, or quadrants An angle can be located in the first, second, third and fourth quadrant, depending on which quadrant contains its terminal side When the angle is between and, the angle is a third quadrant angle Since is between and, it is a thrid quadrant angle2 If drawn in the standard position, which of the following angles terminates in the third quadrant?


Co Ordinates In All 4 Quadrants Ppt Download


Reference Angle Calculator Definition Graph Quadrants
3 Sketch the reference angle in the appropriate quadrant Use the diagram to determine the measure of the related angle in standard position Example 1 – Solve an Angle Given Its Exact Sine, Cosine or Tangent Value Solve for θ a) 𝑖 𝜃=05, 0°≤𝜃Note that in each case, all that really mattered was the sixtydegree triangle in the quadrant where the angle terminated, and the x and yvalues in that quadrantThe fact that you can't draw a right triangle with a base angle of 300° was irrelevant To find the trig ratios, you needed only to draw the terminal side of the angle in whatever quadrant it happened to land, construct a rightWhen angle a is in Quadrant 3 (between 180° and 270°), both the adjacent and the opposite side are negative Hence, Sine and Cosine are negative and since Tangent (T) is a division between two negative numbers, it is the only trigonometric function that is positive Sine, Cosine and Tangent in Quadrant 4


Signs Of Sin Cos Tan In Different Quadrants Finding Value Of Trign
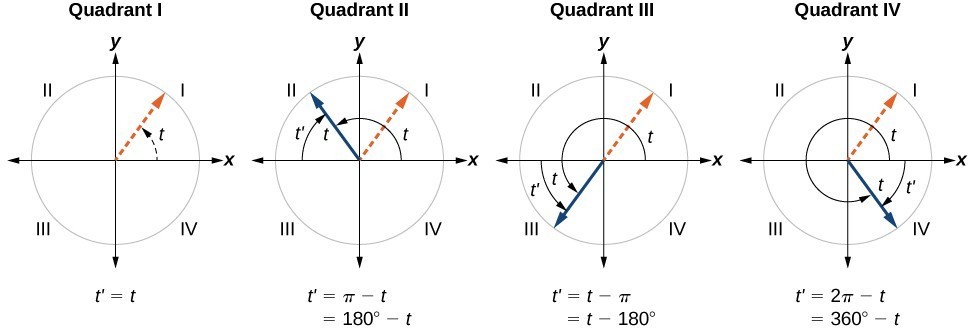


Section 4 4 Reference Angles Precalculus
Nov 23, 16 · cos(A) = −√1 − (2 3)2 cos(A) = −√1 − 4 9 cos(A) = −√ 5 9 cos(A) = − √5 3 Given cos(B) = 3 4 We will need the sin(B) so use the identity sin(B) = ± √1 −cos2(B) We are given that B is in the 4th quadrant, therefore, we chose the negative value for the sine sin(B) = −√1 − cos2(B)Write a Matlab function quadrant that returns the quadrant (1,2,3, or 4) of a list of angles in degrees Assume 90 degrees is quadrant 2, 180 is quadrant 3, 270 is quadrant 4, etc Example quadrant(45) ans=1 quadrant(700 100) ans=4 3The product is (–2) × (–1) = 2, which is positive Any other product in Quadrant III will be positive also, so Quadrant III is not part of my answer In Quadrant IV, I'll pick, say, (3, –4) The product is 3 × (–4) = –12, which is negative Any other product in Quadrant IV will be negative also, so Quadrant IV is part of my answer



Determining Rotations Video Rotations Khan Academy


Unit Circle Wyzant Lessons
The basic geometry rule for finding the quadrant of the coordinate points are 1) If both coordinates are positive, then the point lies in first quadrant 2) If x is negative and y is positive, then the point lies in second quadrant 3) If both coordinates are negative, then the point lies in third quadrant 4) If is x is positive and y is1 Find the reference angle for the given angle 2 Rewrite the original trig function with the reference angle 3 Affix the appropriate sign to the trig function ( or ), depending which quadrant the angle terminates in 4 Evaluate the trig function Evaluating Trigonometric Functions Using Reference Angles Show Stepbystep Solutions1) −300° 2)−50° 3)280° 4)1030° 7 The terminal side of an angle measuring 4π 5 radians lies in Quadrant 1



Graph Each Integer And Its Opposite On A Number Line Ppt Download
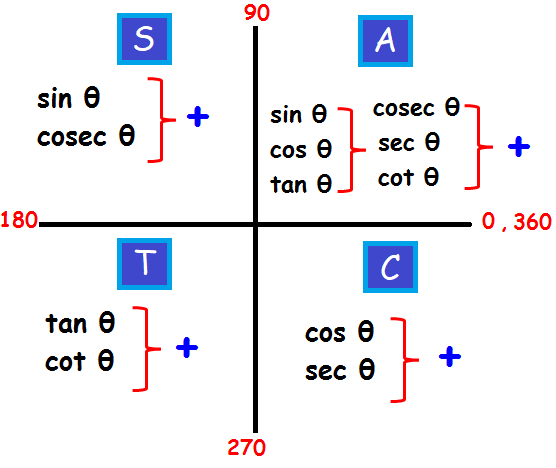


Trigonometry Quadrant Formulas
1 θ=40D 2 160θ= D 3 θ=−3D Exercises Sketch each of the following angles in standard position (Do not use a protractor;⇒ The measures of angles of quadrilateral are 3 6 o, 7 2 o, 1 0 8 o and 1 4 4 o ⇒ We can see measure of all 4 angles are different so, the given quadrilateral is trapezium Answer verified by𝜃=tan−1 @2 −3 A= tan−1 @2 3 A≈ r w { r The value 059 is in quadrant I, so add to place the angle into quadrant III The correct answer is 𝜃= w { u s v= y u radians (If x ⁄is positive and y is negative (in Quadrant 4), then is negative, so that 𝜃=arctan ⁄ ) is in the interval − 2 Q𝜃 Q r (the fourth quadrant)
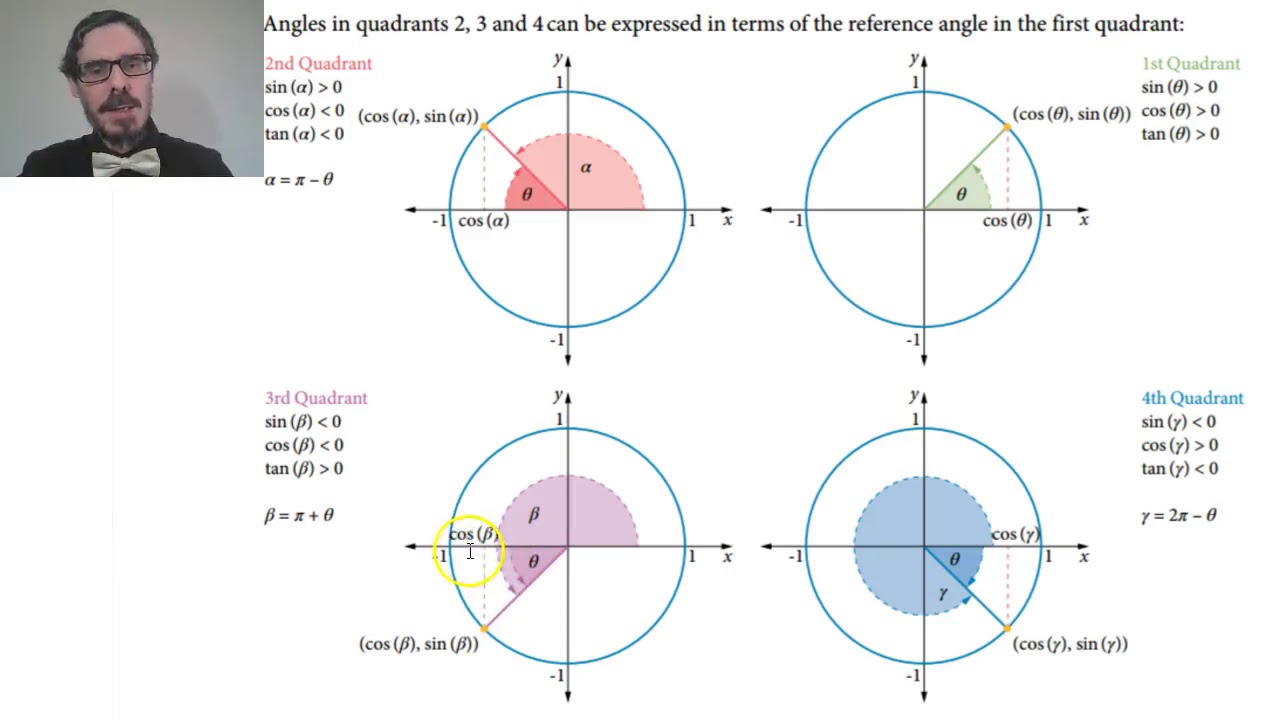


7 1a Reference Angles And 4 Quadrants Youtube



Unit Circle Memorizing The First Quadrant Video Lesson Transcript Study Com
Reverse the order and you have pi/6, pi/4, and pi/3 First Quadrant Points Knowing the angles is the first step, but to be able to do calculations, we have to know the x and y coordinates forJust draw a brief sketch) 1 1θ= D 2 45θ=− D 3 130θ=− D 4 θ=270D θ=−90D 6 θ=750D x y θ Notice that the terminal sides in examples 1 and 3 are in the same position, but they do not represent the same angle (becauseThe given angle may be in degrees or radians Use of calculator to Find the Quadrant of an Angle 1 Enter the angle in Degrees top input example 1250 in Radians second input as a fraction of π Example 27/5 π or 12 π then press the button "Find Quadrant" on the same row If you enter a quadrantal angle, the axis is displayed



Rectangular Coordinate System


Quadrant Plane Geometry Wikipedia
Asked Apr 30, 13 in TRIGONOMETRY by linda Scholar trignometricratios;Sep 01, · There are 2 pi radians in a circle (2 * ) That equates to 6 complete radians and a little more than a quarter of a radian () The length of each arc (s) subtended by an angle of one radian is equal to the radius itselfMay 29, 18 · Transcript Let s see the angles in different Quadrants In Quadrant 1, angles are from 0 to 90 In Quadrant 2, angles are from 90 to 180 In Quadrant 3, angles are from 180 to 270 In Quadrant 4, angles are from 270 to 360 To learn sign of sin, cos, tan in different quadrants, we remember Add Sugar To Coffee Representing as a table Quadrant I Quadrant II Quadrant III Quadrant
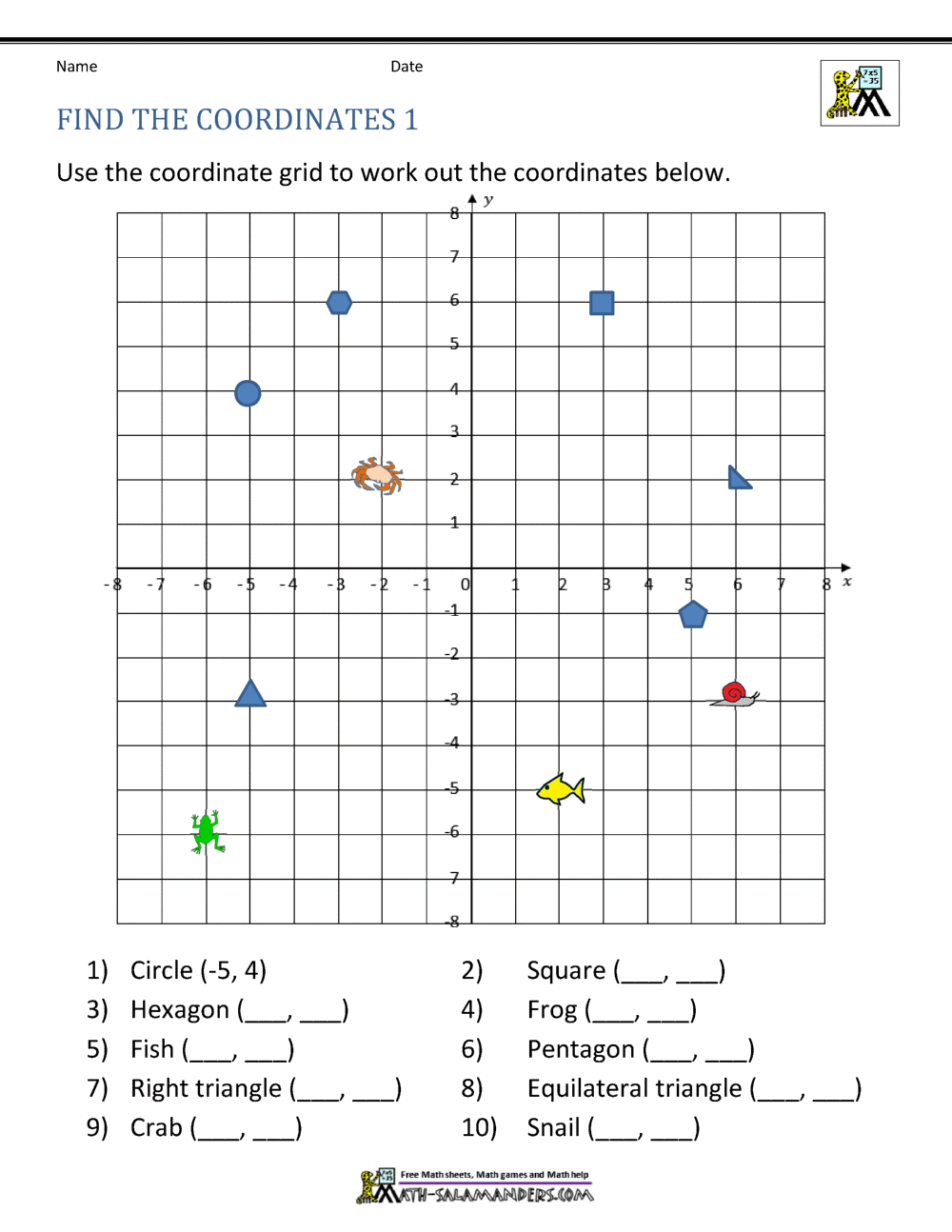


Coordinate Plane Worksheets 4 Quadrants
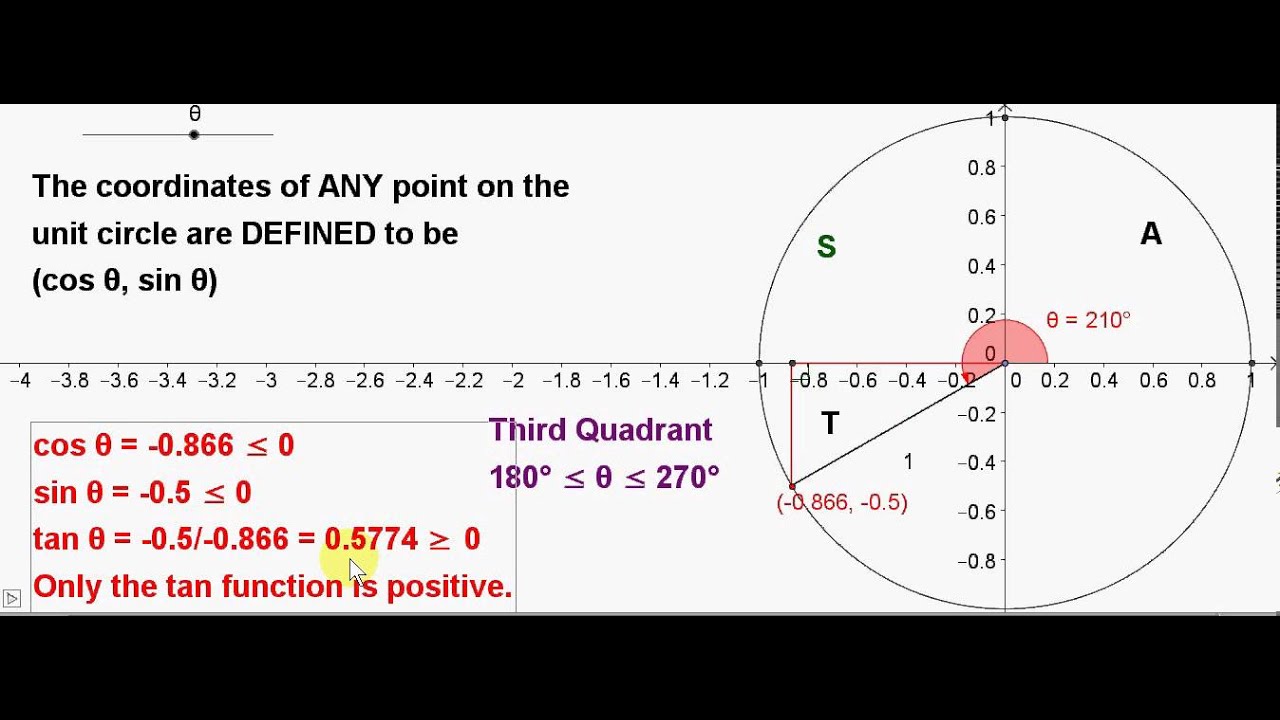


Unit Circle Angles In The Third Quadrant Youtube
The letters ASTC signify which of the trigonometric functions are positive, starting in the top right 1st quadrant and moving counterclockwise through quadrants 2 to 4 Quadrant I (angles from 0 to 90 degrees, or 0 to π/2 radians) All trigonometric functions are positive in this quadrant• If 180° < θ < 270°, the angle is in quadrant 3 • If 270° < θ < 360°, the angle is in quadrant 4 2 For angles in quadrants 24, determine the related acute angle β Using a calculator, determine what the value of the desired ratio would be for angle β 3 The desired ratio for angle θ will be the value obtained from step 2, butIf the terminal arm of an angle falls in quadrants 2, 3 or 4, we use a reference angle of 6;
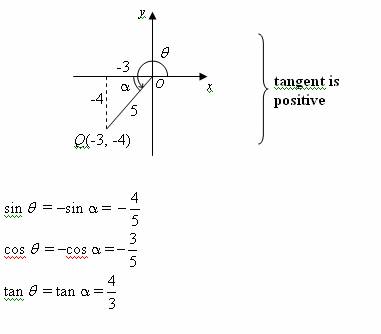


Trigonometric Ratios Solutions Examples Videos
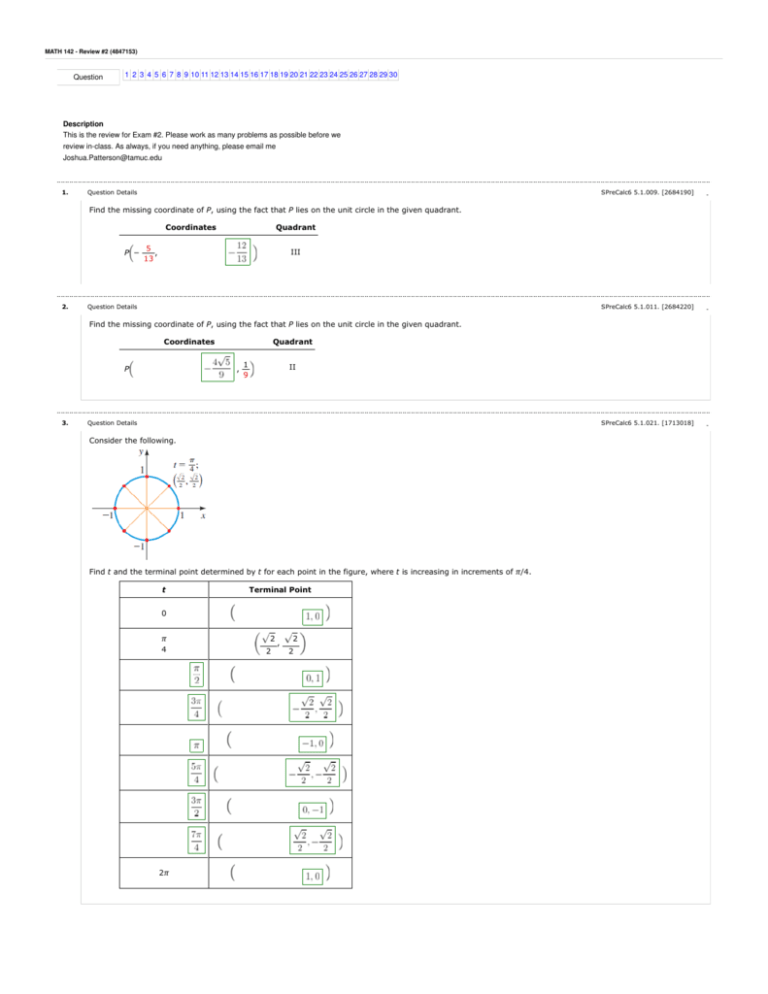


Exam 2 Review Key
If `sin"A"/3 = sin"B"/4 = 1/5` and A, B are angles in the second quadrant then prove that 4cosA 3cosB = – 5 Advertisement Remove all ads Solution Show Solution3 Radians to Quadrants = 90 Radians to Quadrants = 4 Radians to Quadrants = 100 Radians to Quadrants = 5 Radians to Quadrants = 311 0 Radians to Quadrants = 6 Radians to Quadrants = 300 Radians to Quadrants = 7 Radians to Quadrants = 400 Radians to Quadrants = 8



Pdf Half Angles Sequence Of Pi Integral Formulas



Grade 4 Geometry Worksheets Plot Points On A Coordinate Grid K5 Learning
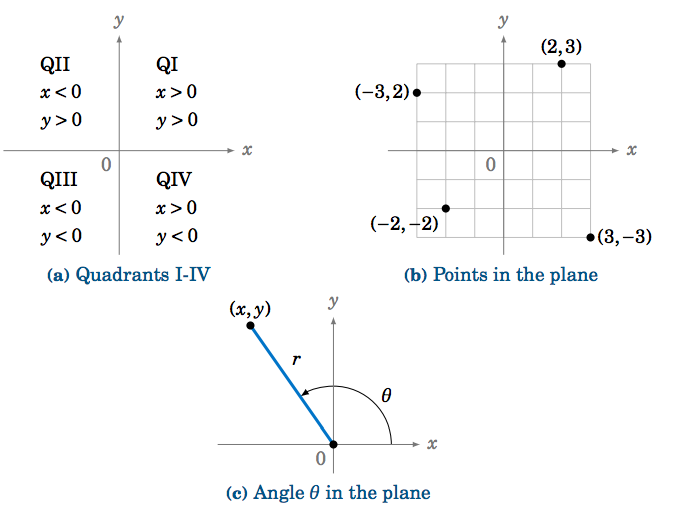


1 4 Trigonometric Functions Of Any Angle Mathematics Libretexts
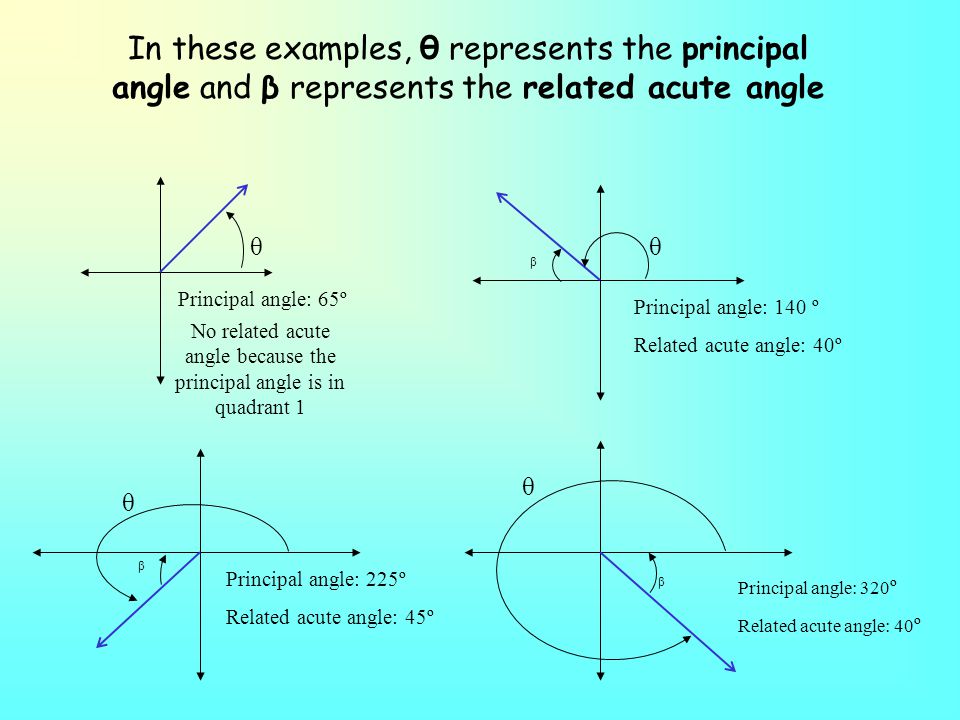


How Do You Find The Related Acute Angle Example
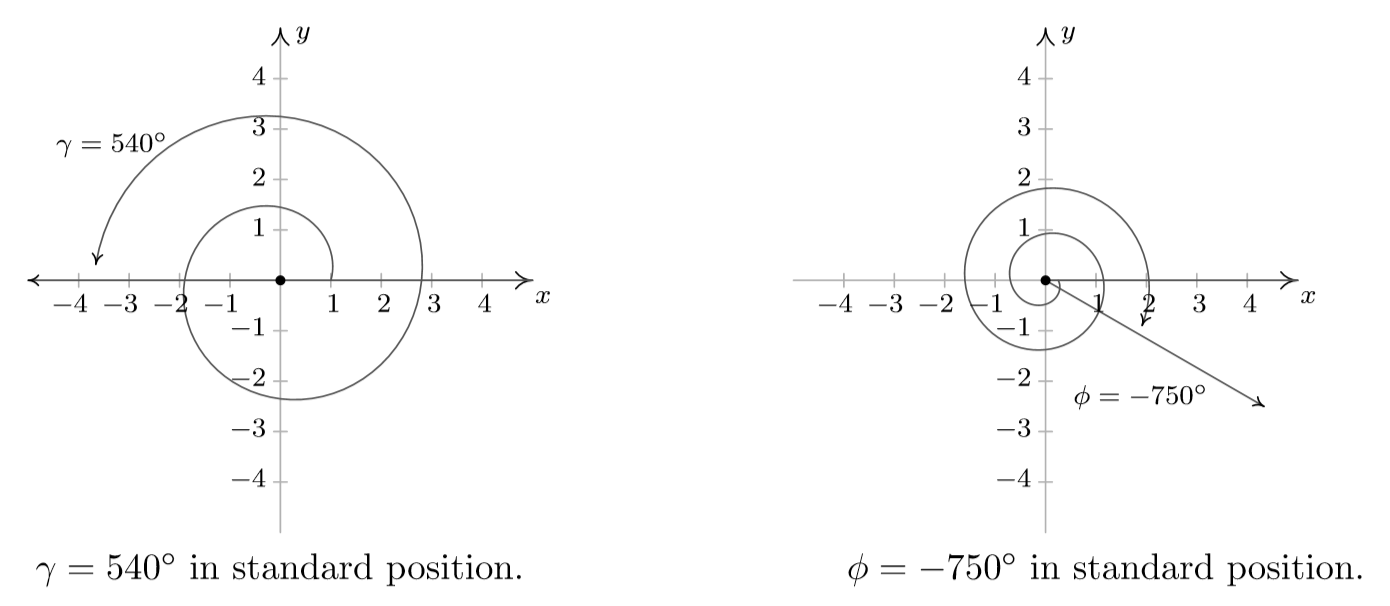


10 1 Angles And Their Measure Mathematics Libretexts


Unit Circle Trigonometry
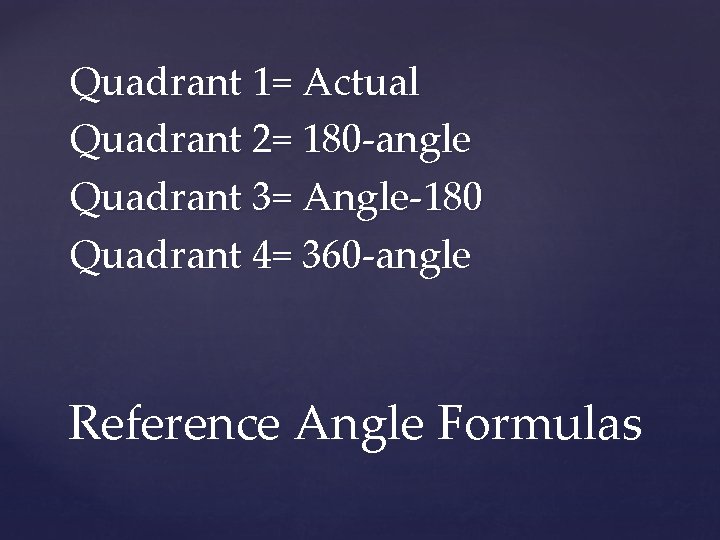


Reference And Coterminal Angles These Are Two Or


Trigonometric Functions Of A General Angle



4 2 Trigonometric Functions Of Acute Angles Manualzz



The Unit Circle Ck 12 Foundation
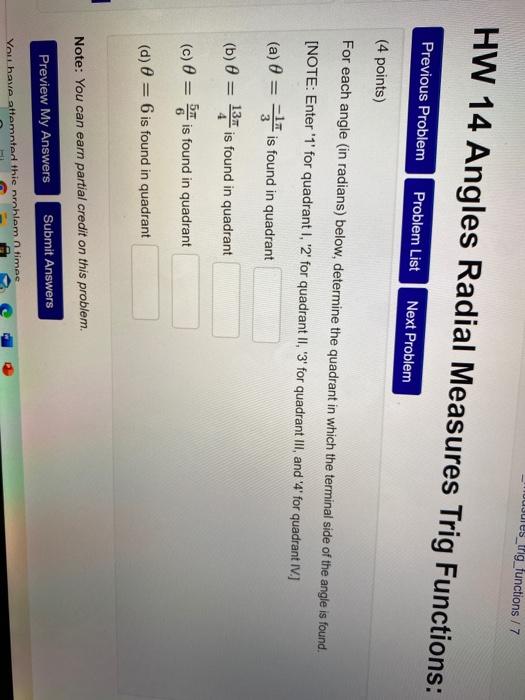


Solved Mujulestrig Functions 7 Hw 14 Angles Radial Meas Chegg Com
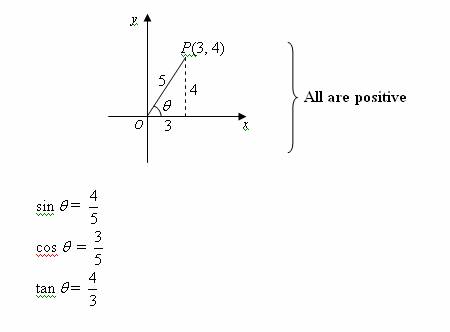


Trigonometric Ratios Solutions Examples Videos
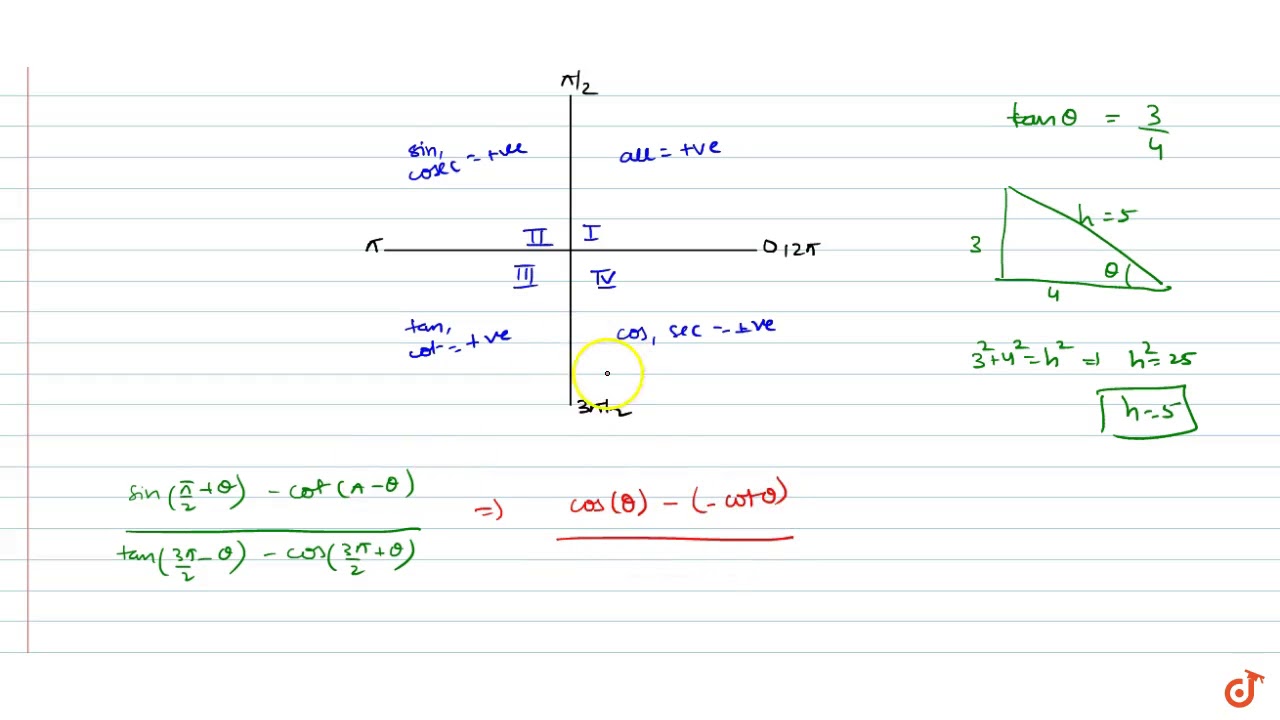


If Tan Theta 3 4 And Theta Is Not In First Quadrant Then Sin Pi 2 Theta Cot Pi Theta Youtube



Exercise 12 4 Page 352 Assignment 2 Solutions Exercise 12 30 40j 30 40 40 30 40 50 Tan Tan 53 306 30 50 306 50 Cos306 Jsin306 To Graph Plot Point 30 40 In 4th Studocu



What Comes Next Here Is A Sequence Of Letters Ppt Video Online Download



Rectangular Coordinate System
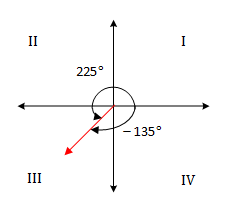


Trigonometry Quadrants And Quadrantal Angles
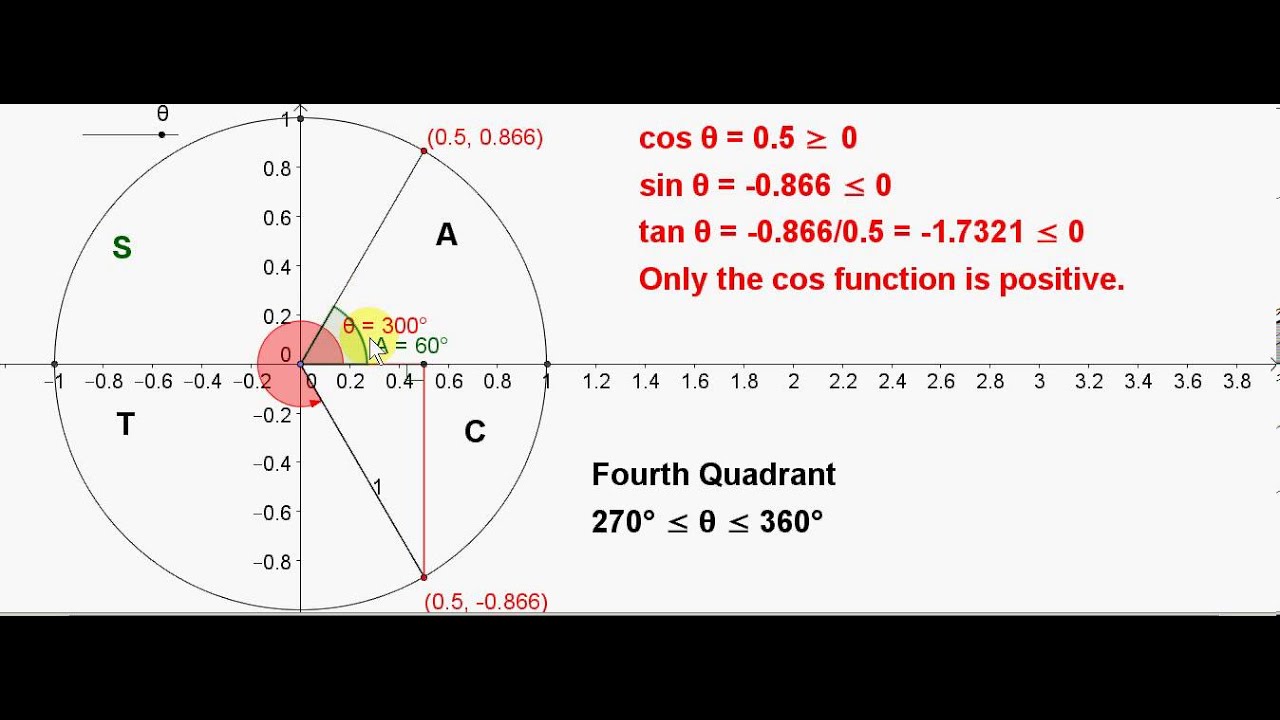


Unit Circle Angles In The Fourth Quadrant Youtube


Year 10a Chapter 04 Introducing The Unit Circle Activity Builder By Desmos



Reference Angle Calculator Definition Graph Quadrants


Content Radian Measure



Identify The Quadrant Of A Point On The Coordinate Plane Youtube



Rotating Shapes About The Origin By Multiples Of 90 Article Khan Academy


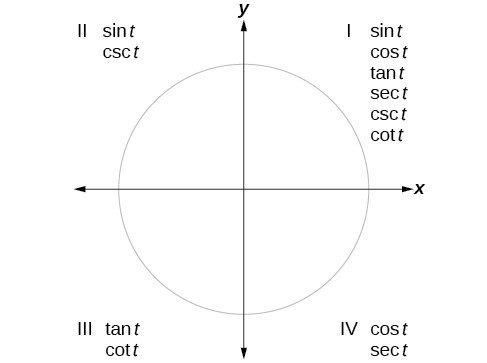
Sine Cosine And Tangent In Four Quadrants



Trigonometric Circle Used To Convert The Angles From Quadrants 2 3 And Download Scientific Diagram



The Unit Circle Ck 12 Foundation


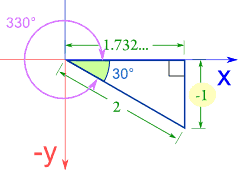
Graph The Oriented Angle In Standard Position 2 1 1 Chegg Com


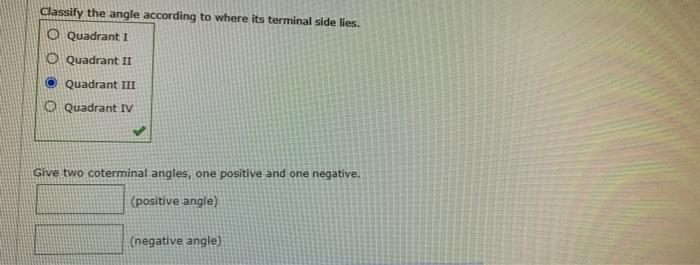
Reference Angle Calculator Pi Day


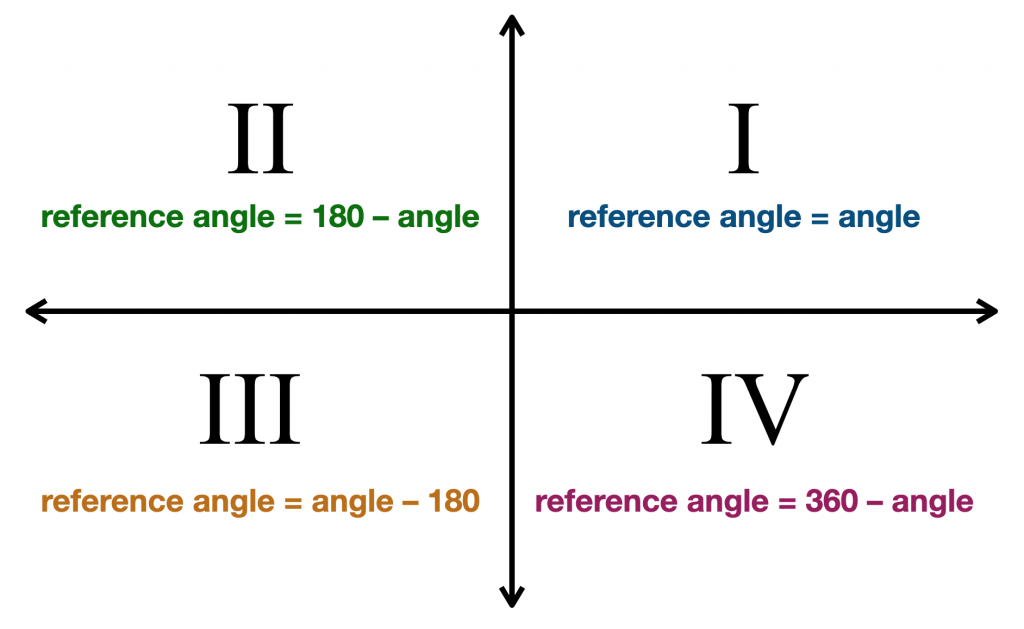
Section 4 4 Reference Angles Precalculus


Unit Circle Wyzant Lessons



Solved 2 Find The Measures Of Angles 1 2 3 And 4 Giv Chegg Com
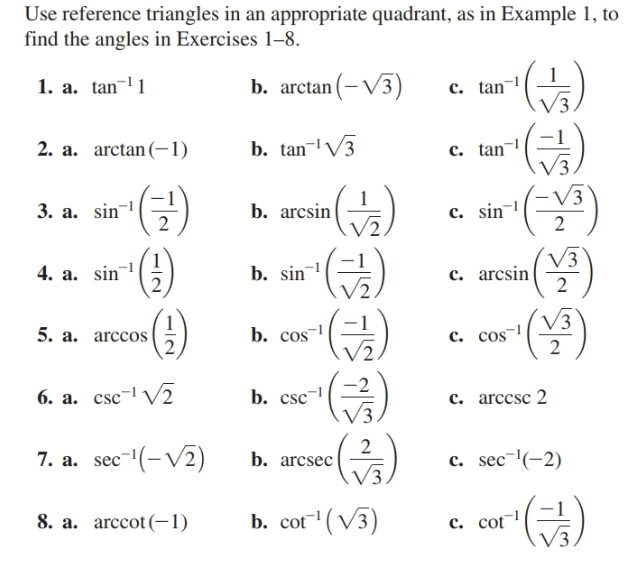


Answered Use Reference Triangles In An Bartleby


Trigonometry Quadrants And Quadrantal Angles



Angles In Different Quadrants Trigonometry
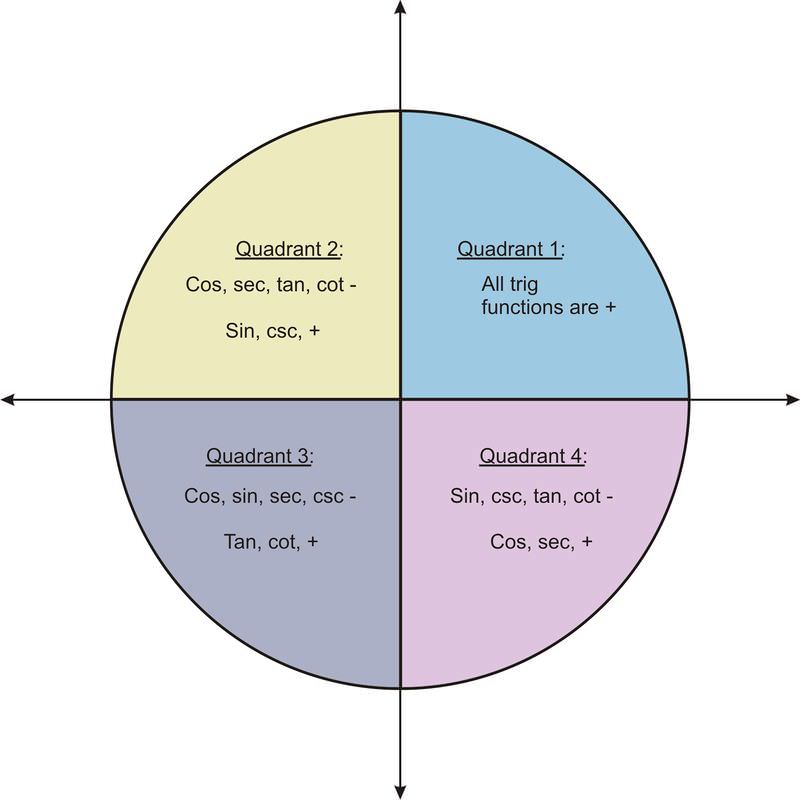


Domain Range And Signs Of Trigonometric Functions Read Trigonometry Ck 12 Foundation


The Coordinate Plane



Points On The Cartesian Plane Ck 12 Foundation
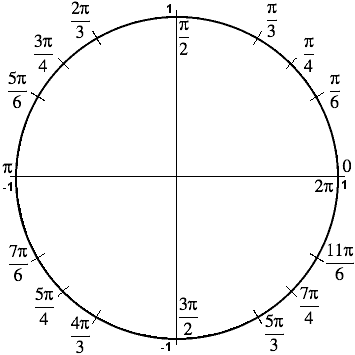


Trigonometry Facts The Amazing Unit Circle
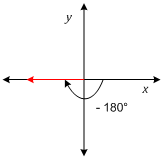


Trigonometry Quadrants And Quadrantal Angles
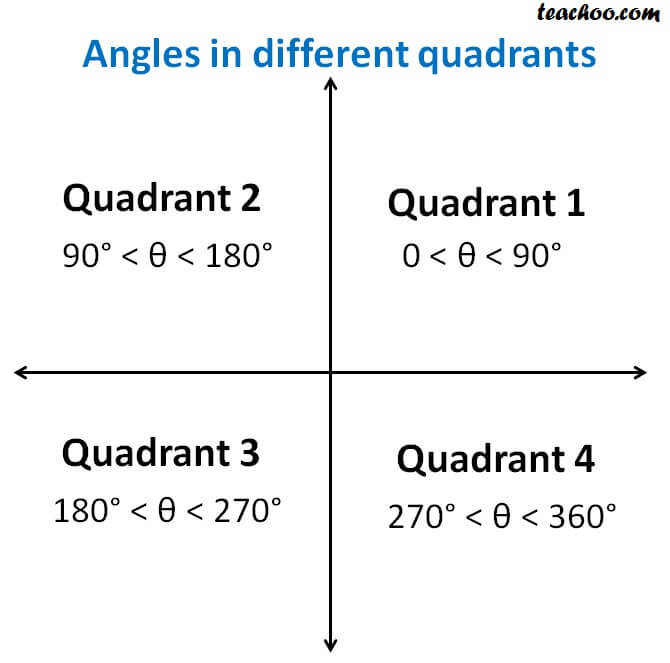


Signs Of Sin Cos Tan In Different Quadrants Finding Value Of Trign


Coordinate Worksheets



Content The Four Quadrants
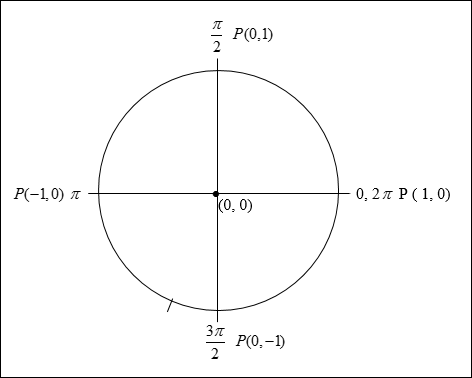


Circular Trigonometry
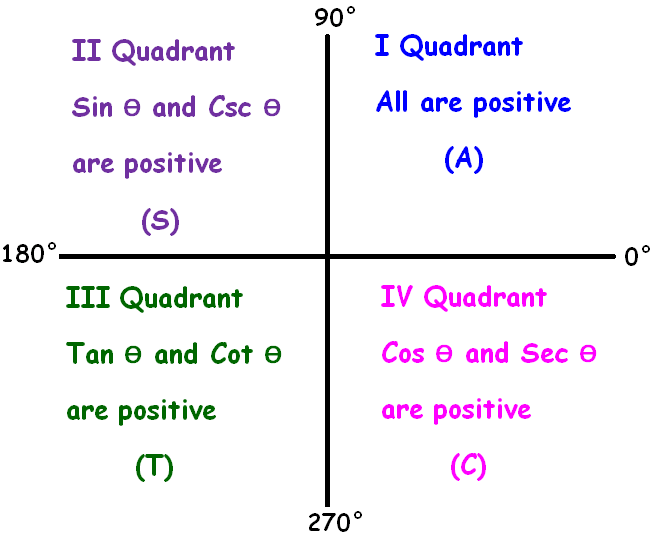


Astc Formula


The Trigonometry Functions
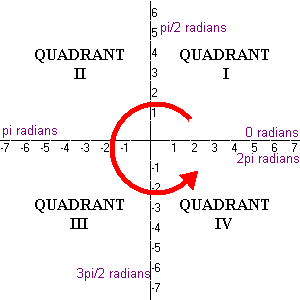


How Do You Determine The Quadrant In Which 6 02 Radians Lies Socratic



Rectangular Coordinate System



Trig Angles And Rotation
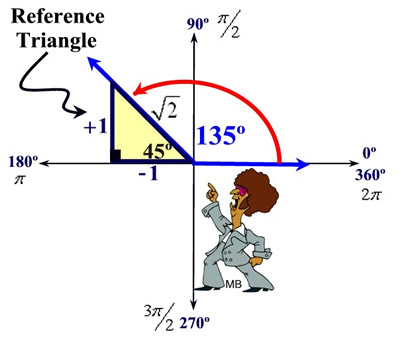


Reference Triangles Mathbitsnotebook Ccss Math



Proper Sheet Of The Plane With Four Quadrants 1 2 3 And 4 Limited Download Scientific Diagram
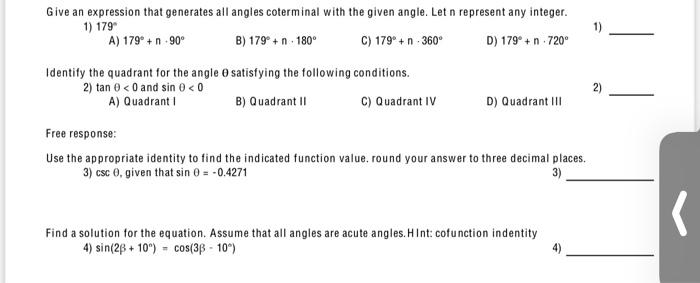


Solved 1 2 Give An Expression That Generates All Angles Chegg Com
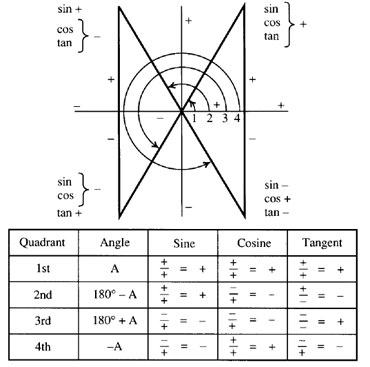


Trigonometric And Geometric Conversions Sin A B Sin A B Sin Ab


Unit Circle Wyzant Lessons



Find Reference Angle


Section 4 3 Precalculus Final


Special Angles In The Unit Circle
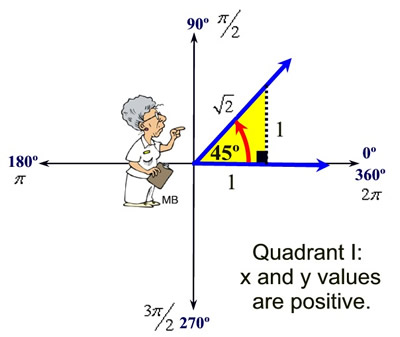


Reference Triangles Mathbitsnotebook Ccss Math


Unit Circle Trigonometry


Coordinates And Graphs Of Lines



Argument Of A Complex Number In Different Quadrants
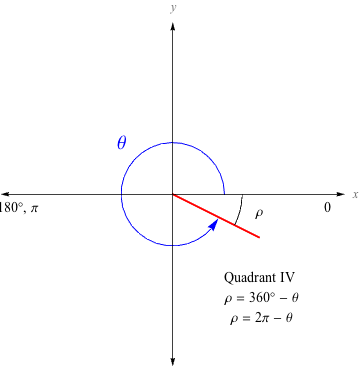


Quadrants



Content The Four Quadrants
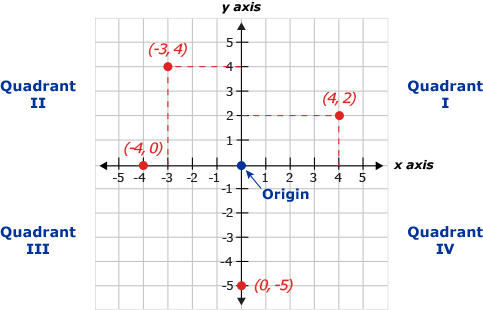


I Pathways Learning Pathways In Adult Education
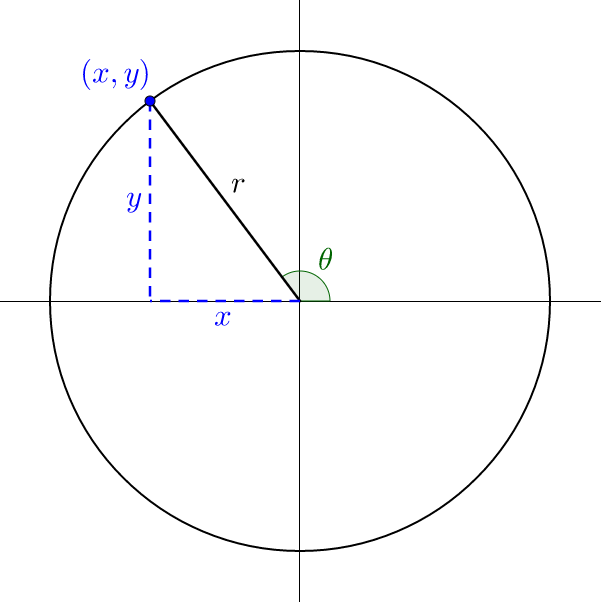


4 05 Trigonometric Functions Of Any Angle



The Unit Circle First Quadrant Angles
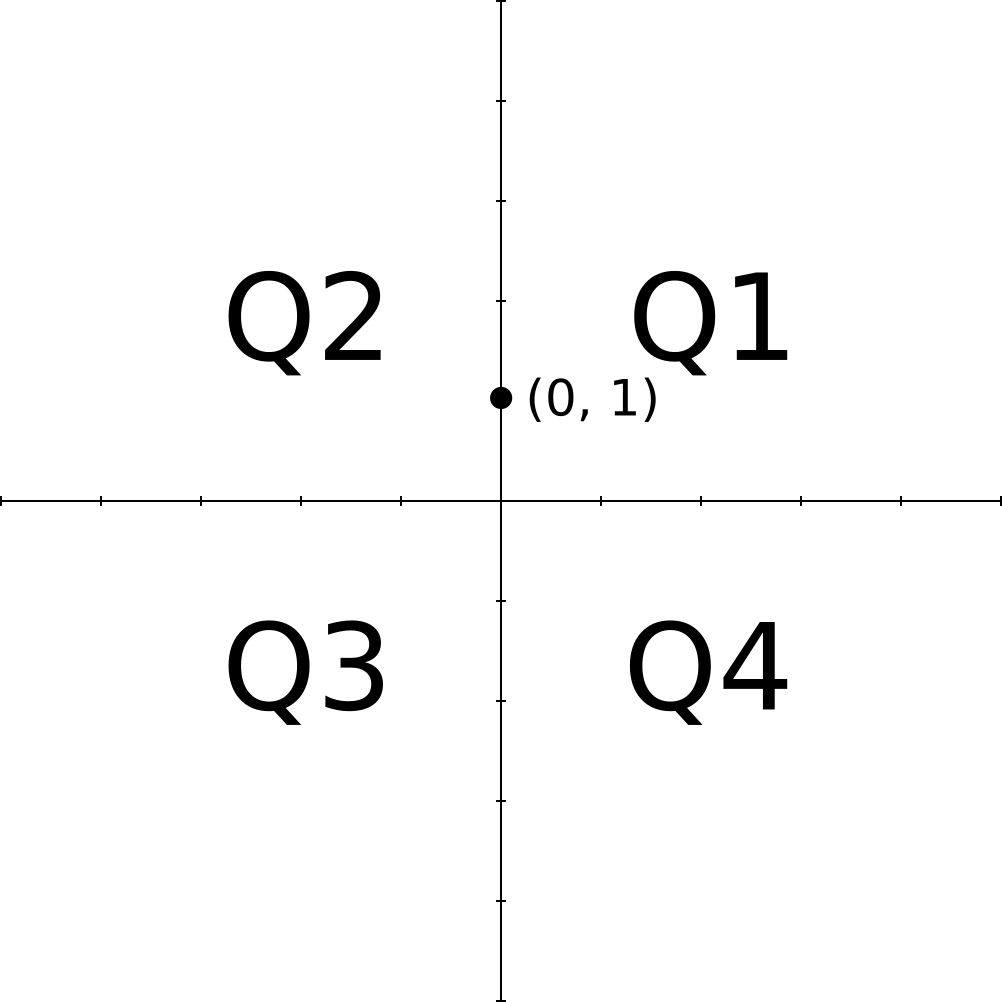


What Quadrant Is The Coordinate 0 1 In Socratic


In What Quadrant Does The Angle 0 Degrees Lie Quora
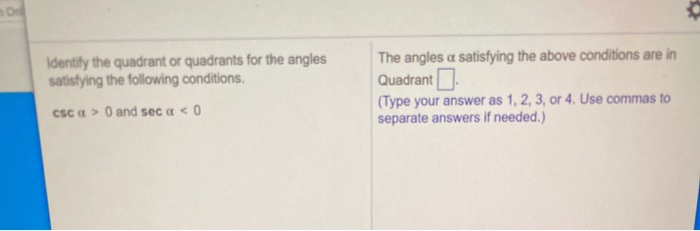


Solved Identify The Quadrant Or Quadrants For The Angles Chegg Com



5 Signs Of The Trigonometric Functions



0 件のコメント:
コメントを投稿